This page shows you the types of questions that are covered on the prealgebra section of the Compass mathematics test.
You will find practice prealgebra problems in each section on this page.
The solutions to the prealgebra problems are provided below the questions in each section.
Calculations with Integers
Integers are real whole numbers.
A question on this part of the test might look like the one below.
Problem: 8 × 5 + 42 ÷ 7 = ?
Solution: For problems like this, you have to divide and multiply first, before you do the addition and subtraction.
8 × 5 = 40
42 ÷ 7 = 6
Then add these together to get the final result.
40 + 6 = 46
Calculations with Positive Integer Exponents
"Positive integer exponents" means problems that contain base numbers and exponents.
Problem: What is 53?
The base number is the big number at the bottom and the exponent is the smaller number at the top right.
In this problem, 5 is the base number and 3 is the exponent.
Solution: When you see an exponent, you have to multiply the base number by itself for the amount of times indicated in the exponent.
In other words, you have to multiply 5 three times.
So 53 = 5 × 5 × 5
5 × 5 × 5 = 25 × 5 = 125
Square Roots and Scientific Notation
Square root problems on this part of the text can look something like this one.
Problem: Simplify the following.
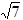 +
+ 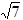
Solution: In order to solve square root problems like this, you need to multiply the square roots for the amount of times they are shown.
In the present problem, the square root of 7 is shown two times.
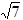 +
+ 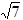 =
=
2 × 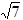
Calculations with Fractions
Problems on this part of the prealgebra test cover skills like finding the lowest common denominator, simplifying fractions and mixed numbers, and multiplying and dividing fractions.
Problem: A job is shared by 4 employees, W, X, Y, and Z.
Employee W works 1/6 of the total hours.
Employee X works 1/3 of the total hours.
Employee Y works 1/2 of the total hours.
What fraction of the remaining hours will employee Z have to work?
Solution: First of all, you have to find the lowest common denominator, also known as the LCD.
Finding the LCD means that you have to make all of the numbers on the bottoms of the fractions the same. The largest existing denominator in this question is 6, so 6 is the lowest common denominator.
The other denominators are 3 (from 1/3 for Employee X) and 2 (from 1/2 for Employee Y).
So, the LCD for Employee X is 3 × 2 = 6
For Employee Y it's 2 × 3 = 6
The sum of the work from all four people must be equal to 100%, simplified to 1.
W + X + Y + Z = 1
1/6 + 1/3 + 1/2 + Z = 1
Now, convert the fraction to the LCD for Employee X.
1/3 × 2/2 = 2/6
Next, find the new fraction for Employee Y.
1/2 × 3/3 = 3/6
Now add the fractions together.
1/6 + 2/6 + 3/6 + Z = 1
6/6 + Z = 1
1 + Z = 1
Z = 0
In other words, there are no hours left for Employee Z to work.
Operations with Decimals
This area includes skills such as converting decimals to fractions and converting fractions to decimals. Here is a sample problem.
Problem: A group of volunteers are collecting food supplies for a project. They need 120 pounds of foodstuff in total for the project.
Volunteer A has collected 273/4 pounds of supplies. Volunteer B has collected 321/5 pounds. Volunteer C has collected 17.35 pounds.
What is remaining amount of food supplies that needs to be collected to complete the project? Provide your answer as a whole number with decimals.
Solution: Convert the fractions to decimals and then add all three amounts together to find the total so far.
Volunteer A: 273/4 = 27.75
Volunteer B: 321/5 = 32.20
27.75 + 32.20 + 17.35 = 77.30
So far, they have collected 77.30 pounds of foodstuff.
Finally, you have to subtract this result from the total amount of 120 for the project in order to find out how many pounds they still need.
120 − 77.30 = 42.70
Calculating Percentages, Ratios, and Proportions
These types of problems often involve fractions. For example:
Problem: Find the value of x that solves the following proportion.
12/8 = x/16
Solution: Simplify the first fraction.
12/8 ÷ 4/4 = 3/2
Then divide the denominator of the second fraction (which is x/16) by the denominator of the simplified first fraction (which is 3/2).
16 ÷ 2 = 8
Now, multiply this result by the numerator of the new first fraction (which is 3/2) to get your result.
8 × 3 = 24
So, 12/8 = 24/16
Finding the Averages
You will need to find averages from the results or data provided in the problem.
Problem: One hundred students took an math exam. The 60 girls in the class had an average score of 80, and the 40 boys in the class had an average of 85. What is the average test score for all of the students?
Solution: Multiply the average for the girls by the number of girls. Then multiply the average for the boys by the number of boys. Then add these two results for the total points for the whole class.
Total points for the girls: 60 × 80 = 4800
Total points for the boys: 40 × 85 = 3400
Total points for entire class: 4800 + 3400 = 8200
When you have got the total points for the class, you divide this by the total number of students to get the class average.
Total number of students: 60 + 40 = 100
8200 ÷ 100 = 82
Now, go on the intermediate algebra problems.
Or skip ahead to the advanced math exercises.
You can find more math practice in the sample of our math download.
